CBSE Class 10 Maths Sample Paper 2022-23 Part 2
CBSE Class 10 Maths Sample Paper 2022-23: Class- X Session- 2022-23 Subject- Mathematics (Standard) Sample Question Paper | CBSE Class 10 Mathematics (Standard) Sample Paper 2022-23: Download CBSE 10 Mathematics (Standard) Sample Question Paper PDF Here
CBSE Class 10 Maths Sample Paper 2022-23: CBSE has released Class 10 Mathematics (Standard) Sample Question Paper. Download direct CBSE Class 10 Mathematics (Standard) Sample Question Paper pdf here. Visit official website at cbse.gov.in or cbseacademic.in.
CBSE Class 10 Mathematics Sample Question Paper 2022-23
General Instruction:
- This Question Paper has 5 Sections A-E.
- Section A has 20 MCQs carrying 1 mark each.
- Section B has 5 questions carrying 02 marks each.
- Section C has 6 questions carrying 03 marks each.
- Section D has 4 questions carrying 05 marks each.
- Section E has 3 case based integrated units of assessment (04 marks each) with sub-parts of thevalues of 1, 1 and 2 marks each respectively.
- All Questions are compulsory. However, an internal choice in 2 Qs of 5 marks, 2 Qs of 3 marksand 2 Questions of 2 marks has been provided. An internal choice has been provided in the
2marks questions of Section E
- Draw neat figures wherever required. Take π =22/7 wherever required if not stated.
CBSE Maths Sample Test Paper for Class X Board Exam 2023 (Part 2)
SECTION – A Questions 1 to 20 carry 1 mark each
1. The value of ‘a’, if HCF (a,18) = 2 and LCM (a,18) = 36, is: (1)
(a) 2 (b) 5 (c) 7 (d) 4
2. The LCM of the smallest two-digit composite number and the smallest composite number is:
(a) 12 (b) 4 (c) 20 (d) 44
3. If r=3 is a root of quadratic equation kr2 – kr–3 = 0, then the value of k is:
(a) 1/2 (b) 3 (c) 1/3 (d) 1/4
4. The solution of the following pair of equations is: x – 3y = 2, 3x – y = 14
(a) x = 5, y = 1 (b) x = 2, y = 3 (c) x = 1, y = 2 (d) x = 1, y = 4
5. What is the positive real root of 64×2 – 1 = 0?
(a) 1/8 (b) 1/4 (c) 1/2 (d) 1/6
7. In ∆ABC and ∆DEF, ∠B = ∠E, ∠F = ∠C, and AB = 3DE. Then, the two triangles are
(a) congruent but not similar (b) similar but not congruent
(c) neither congruent nor similar (d) congruent as well as similar
8. If cosec A = 13/12, then the value of 2 sin A – 3 cos A
4 sin A 9 cos A
(a) 4 (b) 5 (c) 6 (d) 3
9. In the given figure, if TP and TQ are tangents to a circle with center O, so that ∠POQ = 110°, then ∠PTQ is
(a) 110° (b) 90° (c) 80° (d) 70°
10. If the angle of elevation of the top of a tower from a point of observation at a distance of 100 m from its base is 45°, then the height of the tower is:
(a) 160 m (b) 100 m (c) 200 m (d) 150 m
11. The ratio in which the x-axis divides the join of (2, -3) and (5, 6) is:
(a) 1: 2 (b) 3: 4 (c) 1: 3 (d) 1: 5
12. If tan θ = 1, then the value of sec θ + cosec θ is:
(a) 3√2 (b) 4√2 (c) 2√2 (d) √2
13. If the area of a circle is numerically equal to twice its circumference, then the diameter of the circle is
(a) 4 units (b) 6 units (c) 8 units (d) 12 units
14. If the perimeter of a circle is equal to that of a square, then the ratio of the area of circle to the area of the square is
(a) 14: 11 (b) 12: 13 (c) 11:14 (d) 13:12
15. The radii of 2 cylinders are in the ratio 2 : 3 and their heights are in the ratio 5 : 3. Then, the ratio of their volumes is:
(a) 19: 20 (b) 20: 27 (c) 18:25 (d) 17:23
16. For the following distribution:
| Class | 0-5 | 6-11 | 12-17 | 18-23 | 24-29 |
| Frequency | 13 | 10 | 15 | 8 | 11 |
the upper limit of the median class is
(a) 18.5 (b) 20.5 (c) 25.5 (d) 17.5
17. If the mean of the following distribution is 2.6, then the value of y is
| Variable | 1 | 2 | 3 | 4 | 5 |
| Frequency | 4 | 5 | y | 1 | 2 |
(a) 3 (b) 8 (c) 13 (d) 24
18. Two different dice are thrown together. The probability of getting the sum of the two numbers less than 7 is:
(a) 5/12 (b) 7/12 (c) 12/5 (d) 3/11
DIRECTION: In question number 19 and 20, a statement of Assertion (A) is followed by a statement of Reason (R). Choose the correct option
19. Assertion: The HCF of two numbers is 9 and their LCM is 2016. If the one number is 54, then the other number is 336.
Reason: The relation between numbers and their HCF and LCM is product of two numbers a, b = HCF (a, b) × LCM (a, b).
(a) Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
(b) Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).
(c) Assertion (A) is true but Reason (R) is false.
(d) Assertion (A) is false but Reason (R) is true.
20. Assertion (A): The value of y is 3 if the distance between the points P(2, -3) and Q(10, y) is 10. Reason (R): The distance between two points is given by
(a) Both Assertion (A) and Reason (R) are true and Reason (R) is the correct explanation of Assertion (A).
(b) Both Assertion (A) and Reason (R) are true but Reason (R) is not the correct explanation of Assertion (A).
(c) Assertion (A) is true but Reason (R) is false.
(d) Assertion (A) is false but Reason (R) is true.
SECTION – B Questions 21 to 25 carry 2 marks each.
21. For what value of k for which the following pair of linear equations has infinitely many solutions: 2x + 3y = 7, (k – 1)x + (k + 2)y = 3k is
22. In the below-left figure, two chords AB and CD intersect each other at the point P.

Prove that (i) ΔAPC ~ ΔDPB (ii) AP. PB = CP. DP OR If in the given above right sided figure, AB || DE and BD || EF, then prove that DC2 = CF x AC
23. Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
24. Evaluate: 3 cos2 60° sec2 30° – 2 sin2 30° tan2 60°.
25. The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes. OR The radii of the two circles are 8 cm and 6 cm respectively. Find the diameter of the circle having area equal to the sum of the areas of the two circles.
SECTION – C Questions 26 to 31 carry 3 marks each.
26. Prove that √5 is an irrational number.
27. What number should be added to the polynomial x2 – 5x + 4 so that 3 is the zero of the polynomial?
28. A part of monthly hostel charges in a college is fixed and the remaining depends on the number of days one has taken food in the mess. When a student ‘A’ takes food for 22 days, he has to pay Rs. 1380 as hostel charges; whereas student ‘B’, who takes food for 28 days, pays Rs. 1680 as hostel charges. Find the fixed charges and the cost of food per day.
OR Meena went to a bank to withdraw Rs 2,000. She asked the cashier to give her Rs. 50 and Rs. 100 notes only. Meena got 25 notes in all. How many notes of Rs. 50 and Rs. 100 she received?
29. In the given figure, OP is equal to the diameter of the circle. Prove that ABP is an equilateral triangle.
31. All the black face cards are removed from a pack of 52 playing cards. The reaming cards are well shuffled and then a card is drawn at random. Find the probability of getting (i) face card (ii) red card (iii) black card.
SECTION – D Questions 32 to 35 carry 5 marks each.
32. Some students planned a picnic. The total budget for food was Rs. 2,000. But 5 students failed to attend the picnic and thus the cost of food for each member increased by Rs. 20. How many students attended the picnic and how much did each student pay for the food? OR
If Zeba was younger by 5 years than what she really is, then the square of her age (in years) would have been 11 more than five times her actual age. What is her age now?
33. Prove that “If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.”
In the figure, find EC if AD/DB = AE/EC using the above theorem.
34. A tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also, find the cost of the canvas of the tent at the rate of Rs. 500 per m2.
OR A rectangular metal block has length 15 cm, breadth 10 cm and height 5 cm. From this block, a circular hole of diameter 7 cm is drilled out.
Find: (i) the volume of the remaining solid and (ii) the surface area of the remaining solid.
35. The distribution below gives the makes of 100 students of a class, if the median makes are 24, find the frequencies ƒ1 and ƒ2
| Marks | 0-5 | 5-10 | 10-15 | 15-20 | 20-25 | 25-30 | 30-35 | 35-40 |
| No. of Students | 4 | 6 | 10 | ƒ1 | 25 | ƒ2 | 18 | 5 |
SECTION – E (Case Study Based Questions) Questions 36 to 38 carry 4 marks each.
36. Case Study – 1 Ram is watching the top and bottom of a lighthouse from the top of the building. The angles of elevation and depression of the top and bottom of a lighthouse from the top of a 60 m high building are 30° and 60° respectively.

Find (i) the difference between the heights of the lighthouse and the building. (ii) the distance between the lighthouse and the building.
OR The ratio of the height of a lighthouse and the length of its shadow on the ground is 3–√ : 1 What is the angle of elevation?
37. Case Study – 2
Saving money is a good habit and it should be inculcated in children from the beginning. A father brought a piggy bank for his son Aditya. He puts one five-rupee coin of his savings in the piggy bank on the first day. He increases his savings by one five-rupee coin daily.
(i) If the piggy bank can hold 190 coins of five rupees in all, find the number of days he can contribute to put the five-rupee coins into it
(ii) Find the total money he saved. OR
If 6 times the 6th term of an A.P., is equal to 9 times the 9th term, find its 15th term.
38. Case Study – 3
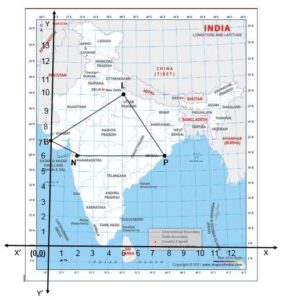
In a GPS, The lines that run east-west are known as lines of latitude, and the lines running north-south are known as lines of longitude. The latitude and the longitude of a place are its coordinates and the distance formula is used to find the distance between two places. The distance between two parallel lines is approximately 150 km. A family from Uttar Pradesh planned a round trip from Lucknow (L) to Puri (P) via Bhuj (B) and Nashik (N) as shown in the given figure below.
Based on the above information answer the following questions using the coordinate geometry.
(i) Find the distance between Lucknow (L) to Bhuj(B).
(ii) If Kota (K), internally divides the line segment joining Lucknow (L) to Bhuj (B) into 3 : 2 then find the coordinate of Kota (K).
(iii) Name the type of triangle formed by the places Lucknow (L), Nashik (N) and Puri (P)
OR Find a place (point) on the longitude (y-axis) which is equidistant from the points Lucknow (L) and Puri (P).
Source: KENDRIYA VIDYALAYA GACHIBOWLI, GPRA CAMPUS
Class- X Session- 2022-23 Subject- Mathematics (Standard) Sample Question Paper | CBSE Class 10 Mathematics (Standard) Sample Paper 2022-23: Download CBSE 10 Mathematics (Standard) Sample Question Paper PDF Here
Read This Too: CBSE Maths Sample Test Paper for Class X – Board Exam 2023
CBSE Sample Papers | CBSE Circulars | Quizzes | Study Material
Join our Premium Telegram and Whatsapp Channel for More News and Updates
For the Latest Educational News (CBSE, ICSE, and State Board News) and live news updates, like us on Facebook or follow us on Twitter and Join our Premium Telegram Channel. Read more on Latest Exams & Results News on Shikshapress.com.